Coupled mode theory#
Caution
This example is under construction, results are not yet all correct
https://www.fiberoptics4sale.com/blogs/wave-optics/coupled-mode-theory https://www.fiberoptics4sale.com/blogs/wave-optics/two-mode-coupling
Show code cell source
from collections import OrderedDict
from itertools import chain
import matplotlib.pyplot as plt
import numpy as np
from scipy.constants import epsilon_0, speed_of_light
from scipy.integrate import solve_ivp
from shapely.geometry import Polygon
from skfem import Basis, ElementTriP0, Mesh
from skfem.io import from_meshio
from femwell.maxwell.waveguide import compute_modes
from femwell.mesh import mesh_from_OrderedDict
Let’s set up the geometry! It’s the cross-section of two parallel waveguides with different widths:
Show code cell source
w_sim = 4
h_clad = 1
h_box = 1
w_core_1 = 0.45
w_core_2 = 0.46
gap = 0.4
h_core = 0.22
offset_heater = 2.2
h_heater = 0.14
w_heater = 2
wavelength = 1.55
k0 = 2 * np.pi / wavelength
polygons = OrderedDict(
core_1=Polygon(
[
(-w_core_1 - gap / 2, 0),
(-w_core_1 - gap / 2, h_core),
(-gap / 2, h_core),
(-gap / 2, 0),
]
),
core_2=Polygon(
[
(w_core_2 + gap / 2, 0),
(w_core_2 + gap / 2, h_core),
(gap / 2, h_core),
(gap / 2, 0),
]
),
clad=Polygon(
[
(-w_sim / 2, 0),
(-w_sim / 2, h_clad),
(w_sim / 2, h_clad),
(w_sim / 2, 0),
]
),
box=Polygon(
[
(-w_sim / 2, 0),
(-w_sim / 2, -h_box),
(w_sim / 2, -h_box),
(w_sim / 2, 0),
]
),
)
resolutions = dict(
core_1={"resolution": 0.03, "distance": 1},
core_2={"resolution": 0.03, "distance": 1},
)
mesh = from_meshio(
mesh_from_OrderedDict(polygons, resolutions, filename="mesh.msh", default_resolution_max=0.2)
)
mesh.draw().show()
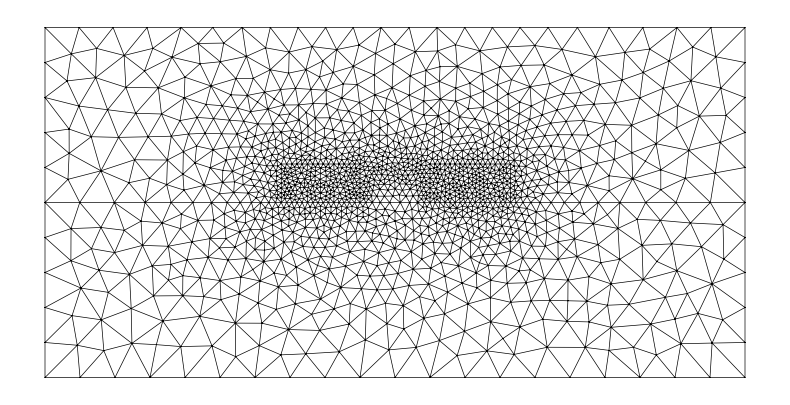
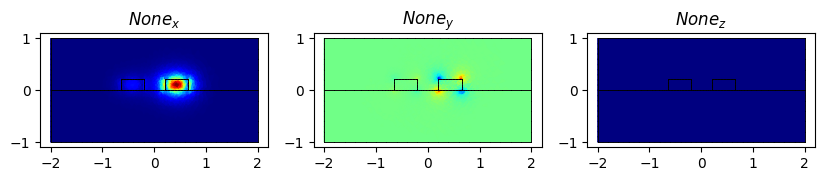
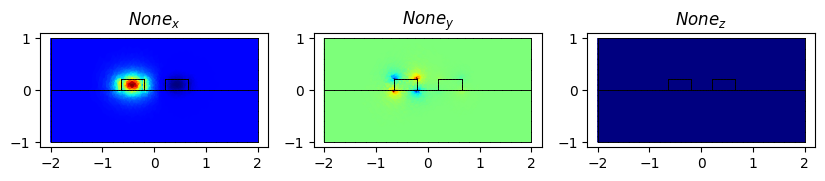
First we plot the symmetric and the asymmetric modes of the geometry with both waveguides:
basis0 = Basis(mesh, ElementTriP0(), intorder=4)
epsilon = basis0.zeros() + 1.444**2
epsilon[basis0.get_dofs(elements=("core_1", "core_2"))] = 3.4777**2
# basis0.plot(epsilon, colorbar=True).show()
modes_both = compute_modes(basis0, epsilon, wavelength=wavelength, mu_r=1, num_modes=2)
modes_both[0].show(modes_both[0].E.real, direction="x")
modes_both[1].show(modes_both[1].E.real, direction="x")
print(
"Refractive index of symmetric and assymetric mode:",
modes_both[0].n_eff,
", ",
modes_both[1].n_eff,
)
# https://www.fiberoptics4sale.com/blogs/wave-optics/directional-couplers
print(
f"Maximum power transfer after {np.pi / (2 * np.pi / wavelength * np.real(modes_both[0].n_eff - modes_both[1].n_eff))} um prop length"
)
/tmp/ipykernel_9110/3693127342.py:7: DeprecationWarning: The behavior of passing an array directly to `show` is deprecated and will be removed in the future. Use `plot` instead.
modes_both[0].show(modes_both[0].E.real, direction="x")
/tmp/ipykernel_9110/3693127342.py:8: DeprecationWarning: The behavior of passing an array directly to `show` is deprecated and will be removed in the future. Use `plot` instead.
modes_both[1].show(modes_both[1].E.real, direction="x")
Refractive index of symmetric and assymetric mode: (2.3711213710792856+0j) , (2.349170333123299+0j)
Maximum power transfer after 35.30584756647668 um prop length
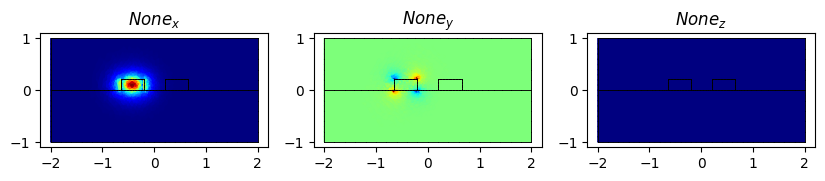
And then we plot the modes of each waveguide while setting the shape of the other one to oxide
epsilon = basis0.zeros() + 1.444**2
epsilon[basis0.get_dofs(elements="core_1")] = 3.4777**2
# basis0.plot(epsilon, colorbar=True).show()
modes_1 = compute_modes(basis0, epsilon, wavelength=wavelength, mu_r=1, num_modes=1)
print("Effective refractive index of the mode of the first waveguide", modes_1[0].n_eff)
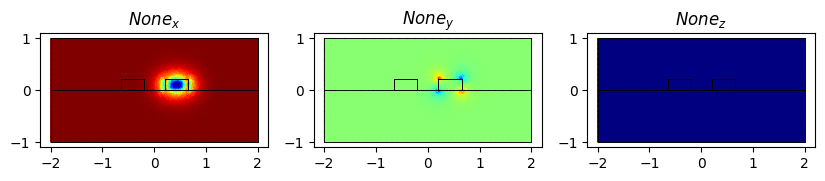
modes_1[0].show(modes_1[0].E.real, direction="x")
epsilon_2 = basis0.zeros() + 1.444**2
epsilon_2[basis0.get_dofs(elements="core_2")] = 3.4777**2
# basis0.plot(epsilon_2, colorbar=True).show()
modes_2 = compute_modes(basis0, epsilon_2, wavelength=wavelength, mu_r=1, num_modes=1)
print("Effective refractive index of the mode of the second waveguide", modes_2[0].n_eff)
modes_2[0].show(modes_2[0].E.real, direction="x")
Effective refractive index of the mode of the first waveguide (2.3495403991911963+0j)
/tmp/ipykernel_9110/185950769.py:6: DeprecationWarning: The behavior of passing an array directly to `show` is deprecated and will be removed in the future. Use `plot` instead.
modes_1[0].show(modes_1[0].E.real, direction="x")
Effective refractive index of the mode of the second waveguide (2.370660339027542+0j)
/tmp/ipykernel_9110/185950769.py:13: DeprecationWarning: The behavior of passing an array directly to `show` is deprecated and will be removed in the future. Use `plot` instead.
modes_2[0].show(modes_2[0].E.real, direction="x")
length = 200
ts = np.linspace(0, length, 1000)
epsilons = [epsilon, epsilon_2]
num_modes = len(modes_1) + len(modes_2)
overlap_integrals = np.zeros((num_modes, num_modes), dtype=complex)
for i, mode_i in enumerate(chain(modes_1, modes_2)):
for j, mode_j in enumerate(chain(modes_1, modes_2)):
overlap_integrals[i, j] = mode_i.calculate_overlap(mode_j)
print("overlap", overlap_integrals)
# plt.imshow(np.abs(overlap_integrals))
# plt.colorbar()
# plt.show()
coupling_coefficients = np.zeros((num_modes, num_modes), dtype=complex)
for i, mode_i in enumerate(chain(modes_1, modes_2)):
for j, mode_j in enumerate(chain(modes_1, modes_2)):
coupling_coefficients[i, j] = (
k0
* speed_of_light
* epsilon_0
* mode_i.calculate_coupling_coefficient(
mode_j, epsilons[(j // len(modes_1) + 1) % 2] - 1.444**2
)
* 0.5
)
print(coupling_coefficients)
# plt.imshow(np.abs(coupling_coefficients))
# plt.colorbar()
# plt.show()
kappas = np.array(
[
[
(
coupling_coefficients[i, j]
- overlap_integrals[i, (i + 1) % 2]
* coupling_coefficients[(i + 1) % 2, j]
/ overlap_integrals[(i + 1) % 2, (i + 1) % 2]
)
/ (
1
- overlap_integrals[0, 1]
* overlap_integrals[1, 0]
/ (overlap_integrals[0, 0] * overlap_integrals[1, 1])
)
for i in range(2)
]
for j in range(2)
]
)
print(kappas)
delta = 0.5 * (
np.real(modes_1[0].n_eff) * k0 + kappas[1, 1] - (np.real(modes_2[0].n_eff) * k0 + kappas[0, 0])
)
print(delta, np.real(modes_1[0].n_eff) * k0, kappas[1, 1])
beta_c = (kappas[0, 1] * kappas[1, 0] + delta**2) ** 0.5
print(np.pi / (2 * beta_c))
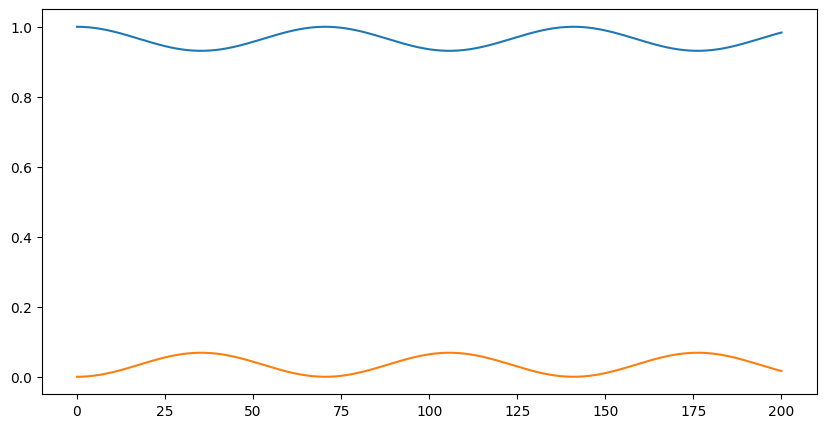
eta = np.abs(kappas[1, 0] ** 2 / beta_c**2) * np.sin(beta_c * 1e3)
print("eta", eta, np.abs(kappas[1, 0] ** 2 / beta_c**2))
plt.plot(ts, 1 - np.abs(kappas[1, 0] ** 2 / beta_c**2 * np.sin(beta_c * ts) ** 2))
plt.plot(ts, np.abs(kappas[1, 0] ** 2 / beta_c**2 * np.sin(beta_c * ts) ** 2))
plt.show()
overlap [[ 1. +0.j -0.01395496+0.j]
[-0.01395496+0.j 1. +0.j]]
[[ 0.00073614+0.j -0.011687 +0.j]
[-0.01287565+0.j 0.00062816+0.j]]
[[ 0.00055657+0.j -0.01286788+0.j]
[-0.01168051+0.j 0.00046516+0.j]]
(-0.04285231595178107+0j) 9.524256590208378 (0.0004651580593850146+0j)
(35.24211803142083+0j)
eta (0.03816703470993108+0j) 0.06867639765289534
see http://home.iitj.ac.in/~k.r.hiremath/research/thesis.pdf , not yet finished
def fun(t, y):
phase_matrix = [
[
np.exp(2j * np.pi / wavelength * (mode_i.n_eff - mode_j.n_eff) * t)
for mode_j in chain(modes_1, modes_2)
]
for mode_i in chain(modes_1, modes_2)
]
matrix = (
np.linalg.inv(overlap_integrals * phase_matrix)
@ (coupling_coefficients * phase_matrix)
* -1j
)
return (matrix @ y).ravel()
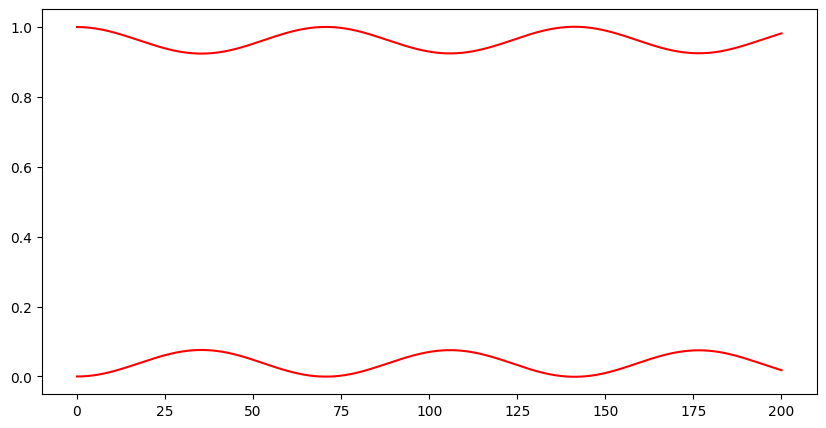
result = solve_ivp(fun, [0, length], np.array((1, 0), dtype=complex), t_eval=ts)
ys = result.y.T
plt.plot(ts, np.abs(np.array(ys)[:, 0]) ** 2, "r")
plt.plot(ts, 1 - np.abs(np.array(ys)[:, 0]) ** 2, "r")
# plt.plot(ts, np.array(ys).imag.reshape((-1,)+matrix.shape)@(1,0), 'g')
plt.show()
two modes#
R = [np.abs(modes_1[0].calculate_overlap(mode_j) ** 2) for mode_j in modes_both]
print(R)
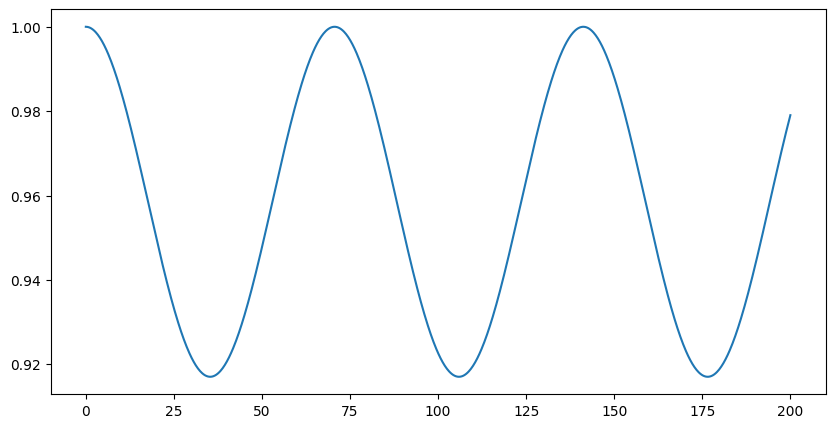
P = (
R[0] ** 2
+ R[1] ** 2
+ 2
* R[0]
* R[1]
* np.cos(2 * np.pi / wavelength * (modes_both[0].n_eff - modes_both[1].n_eff) * ts)
)
plt.plot(ts, P)
plt.show()
[0.02120314990994301, 0.9788107503909889]
/home/runner/miniconda3/lib/python3.12/site-packages/matplotlib/cbook.py:1709: ComplexWarning: Casting complex values to real discards the imaginary part
return math.isfinite(val)
/home/runner/miniconda3/lib/python3.12/site-packages/matplotlib/cbook.py:1345: ComplexWarning: Casting complex values to real discards the imaginary part
return np.asarray(x, float)
plt.plot(ts, P)
plt.plot(ts, 1 - np.abs(kappas[1, 0] ** 2 / beta_c**2) * np.sin(beta_c * ts) ** 2)
plt.plot(ts, np.abs(np.array(ys)[:, 0]) ** 2, "r")
plt.plot(ts, 1 - np.abs(np.array(ys)[:, 0]) ** 2, "r")
# plt.plot(ts, np.array(ys).imag.reshape((-1,)+matrix.shape)@(1,0), 'g')
plt.show()
